January 25, 2018 — Support my next blog post, buy me a coffee ☕.
I have just submitted a paper about nonlocal equations on the sphere with my colleagues Mikael Slevinsky and Qiang Du. Here are the main ideas of our work.
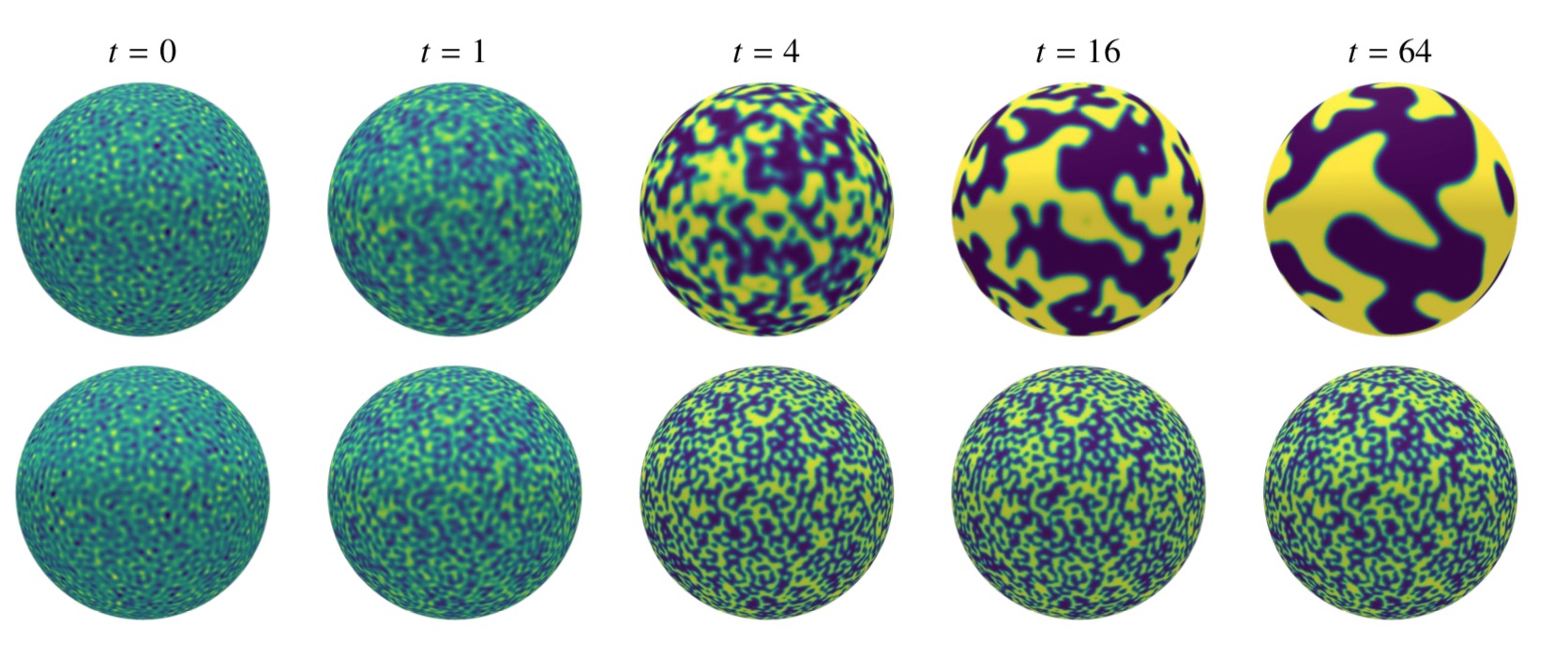
We proposed a fast spectral method for computing solutions of nonlocal models of the form $$ u_t = \epsilon^2\mathcal{L}_\delta u + \mathcal{N}(u), \;\; u(t=0,\boldsymbol{x}) = u_0(\boldsymbol{x}), $$ where \(u(t,\boldsymbol{x})\) is a function of time \(t\ge0\) and position \(\boldsymbol{x}\) on the unit sphere \(\mathbb{S}^2\subset\mathbb{R}^3\), \(\mathcal{N}\) is a nonlinear operator with constant coefficients (e.g., \(\mathcal{N}(u)=u-u^3\)), and \(\mathcal{L}_{\delta}\) is a nonlocal Laplace–Beltrami operator, $$ \mathcal{L}_\delta u(\boldsymbol{x}) = \int_{\mathbb{S}^2} \rho_\delta(\vert\boldsymbol{x}-\boldsymbol{y}\vert) [u(\boldsymbol{y}) - u(\boldsymbol{x})]\,d\Omega(\boldsymbol{y}). $$ In the definition above, \(d\Omega(\boldsymbol{y})\) denotes the standard measure on \(\mathbb{S}^2\) and \(\rho_\delta\) is a suitably defined nonlocal kernel with horizon \(0<\delta\le2\), which determines the range of interactions.
There has been substantial work on the numerical approximation of this type of equations in Euclidean geometries. However, no study has been attempted so far to investigate similar discretizations on the sphere. It is of practical interest to study the extension to non-Euclidean geometries (the sphere being a representative example) for the modeling of anomalous diffusion, pattern formation, and image analysis.
Our algorithms are based on the diagonalizability of nonlocal diffusion operators in the basis of spherical harmonics, the computation of their eigenvalues to high relative accuracy using quadrature and asymptotic formulas, and a fast spherical harmonic transform. The picture at the top shows the numerical solutions of the local (first row) and nonlocal (second row) Allen–Cahn equation. If you want to know more about our algorithms, check our paper out!
Blog posts about spectral methods
2023 Nonlocal vector calculus on the sphere
2020 Exponential integrators for stiff PDEs
2018 Computer-assisted proofs for PDEs
2018 Spherical caps in cell polarization
2018 Solving nonlocal equations on the sphere
2018 Gibbs phenomenon and Cesàro mean
2017 Solving PDEs on the sphere
2017 When planets dance